In the previous section, we saw the basics about bond order. In this section, we will see resonance structures. We will explain it using some examples
Example 1: Resonance structures of ozone
1. Let us first draw the Lewis dot structure of O3 (ozone) molecule
(We have seen the steps in an earlier section 4.2)
Step 1: Finding the number of dots
• Number of valence electrons of O = 6
• So total number of valence electrons = (3 × 6) = 18
Step 2: The skeletal structure
• The skeletal structure is shown in fig.4.44(a) below:
Step 3: Preliminary single bonds
• The three atoms are joined by '─' as shown in fig.4.44(b) above
Step 4: Preliminary distribution of electrons
• First make the two outer O atoms octet
• Then give the remaining electrons to the central O atom
• This is shown in fig.c
• In the fig.c, we see that:
♦ The valence electrons of the outer O atoms are shown in green color
♦ The valence electrons of the central O atom are shown in red color
• Both the outer O atoms have 8 electrons (including the one red dot in the single bonds)
♦ So the number of electrons used up for making those O atoms octet = 16
♦ So the number of remaining electrons = (18-16) = 2
♦ These 2 electrons are given to the central O atom
• There are no more electrons to distribute
Step 5: Check for octet
• Both the outer O atoms have got 8 electrons each. They have attained octet
• The central O atom has got only 6 electrons. It has not attained octet
• Thus, the preliminary distribution needs to be changed
■ Rearrangement:
• In fig.c, take a lone pair from the left side O atom
• Using those two electrons, convert the left side single bond to a double bond
• This is shown in fig.d
• In fig.d, all the atoms have octet. So it is a stable O3 molecule
2. Another possible rearrangement
• We already know how to obtain the structure in fig.4.44(d) above
♦ We obtained it based on fig.4.44(c)
• That same fig.4.44(c) is shown again in fig.4.45(c) below:
• In fig.4.45(c), take a lone pair from the right side O atom
• Using those two electrons, convert the right side single bond to a double bond
• This is shown in fig.4.45(d')
• In fig.d', all the atoms have octet. So it is also a stable O3 molecule
3. So we have two possible structures of O3
♦ One is the structure in fig.4.44(d)
♦ The other is the structure in fig.4.45(d')
• They are shown together in figs.4.46 below:
4. Now the next question arises:
■ In reality, which is the correct form in which O3 exists? Fig.4.46 (d) or (d')?
• The answer can be written in 5 steps:
(i) An O-O single bond will have a length of 148 pm.
♦ This is shown in figs.4.47(a) and (b) below.
(ii) An O=O double bond will have a length of 121 pm
♦ This is shown in figs.4.47(a) and (b) below.
(iii) With this information, we examine an actual O3 molecule.
♦ We would expect the distance between one pair of O atoms to be 148 pm
♦ We would expect the distance between the other pair of O atoms to be 121 pm
(iv) But surprisingly, the actual values are different from both 148 and 121
• In fact there are no 'values'. There is only one value. It is 128 pm
• The distance between atoms in both pairs is 128 pm.
• This is shown in fig.4.47(c) below:
• (a) and (b) are the two possible structures that we saw in fig.4.46
• (c) is different from both (a) and (b)
(v) Note the bonds in fig.c. They are neither single bonds nor double bonds
• This is indicated by the dashed lines
■ Fig.c represents the structure of O3 more accurately
5. The structures in (a) and (b) are called canonical structures
♦ They are also called resonance structures
(For some molecules, there will be more than two resonance structures)
6. Resonance structures are indicated by giving double headed arrows between them
• The structure in (c) is called the hybrid of the resonance structures
♦ It is also called the resonance hybrid
Example 2: Resonance structures of carbon dioxide
1. Let us first draw the Lewis dot structure of CO2 (carbon dioxide) molecule
(We have seen the steps in an earlier section 4.2)
Step 1: Finding the number of dots
• Number of valence electrons of C = 4
• Number of valence electrons of O = 6
• So total number of valence electrons = [4+(2 × 6)] = 16
Step 2: The skeletal structure
• The skeletal structure is shown in fig.4.48(a) below:
Step 3: Preliminary single bonds
• The three atoms are joined by '─' as shown in fig.4.48(b) above
Step 4: Preliminary distribution of electrons
• First make the two outer O atoms octet
• Then give the remaining electrons to the central C atom
• This is shown in fig.c
• In the fig.c, we see that:
♦ The valence electrons of the outer O atoms are shown in green color
♦ The valence electrons of the central O atom are shown in red color
• Both the outer O atoms have 8 electrons (including the one red dot in the single bonds)
♦ So the number of electrons used up for making those O atoms octet = 16
♦ So the number of remaining electrons = (16-16) = 0
• There are no more electrons to distribute
Step 5: Check for octet
• Both the outer O atoms have got 8 electrons each. They have attained octet
• The central C atom has got only 4 electrons. It has not attained octet
• Thus, the preliminary distribution needs to be changed
■ Rearrangement:
• In fig.c, take a lone pair from the left side O atom
♦ Using those two electrons, convert the left side single bond to a double bond
• Again, in fig.c, take a lone pair from the right side O atom
♦ Using those two electrons, convert the right side single bond to a double bond
• This is shown in fig.d
• In fig.d, all the atoms have octet. So it is a stable CO2 molecule
2. Another possible rearrangement
• We already know how to obtain the structure in fig.4.48(d) above
♦ We obtained it based on fig.4.48(c)
• That same fig.4.48(c) is shown again in fig.4.49(c) below:
• In fig.4.49(c), take two lone pairs from the left side O atom
• Using those four electrons, convert the left side single bond to a triple bond
• This is shown in fig.4.49(d')
• In fig.4.49(d'), all the atoms have octet. So it is also a stable CO2 molecule
3. Yet another possible rearrangement
• The above rearrangement in fig.4.49(d') was based on fig.4.48(c)
• That same fig.4.48(c) is shown again in fig.4.50(c) below:
• In fig.4.50(c), take two lone pairs from the right side O atom
• Using those four electrons, convert the right side single bond to a triple bond
• This is shown in fig.4.50(d'')
• In fig.4.50(d''), all the atoms have octet. So it is also a stable CO2 molecule
4. So we have three possible structures of CO2
♦ The structure in fig.4.48(d)
♦ The structure in fig.4.49(d')
♦ The structure in fig.4.50(d'')
• They are shown together in fig.4.51 below:
5. Now the next question arises:
■ In reality, which is the correct form in which CO2 exists? Fig.4.51 (d), (d') or (d'')?
• The answer can be written in 4 steps:
(i) A C-O single bond will have a length of 134 pm
(ii) A C=O double bond will have a length of 121 pm
(iii) A C≡O triple bond will have a length of 110 pm
(iv) With this information, we examine the bond lengths in an actual CO2 molecule
• Surprisingly, the actual values are different from 134, 121 and 110
• In fact there are no 'values'. There is only one value. It is 115 pm
• The distance between C and O atoms in both pairs is 115 pm
6. The structures in figs (d) (d') and (d'') are called canonical structures of CO2
• They are also called resonance structures of CO2
7. Resonance structures are indicated by giving double headed arrows between them
1. Let us first draw the Lewis dot structure of CO32- (carbonate ion)
(We have seen the structure in an earlier section 4.3. But there we did not explore the various possible arrangements)
Step 1: Finding the number of dots
• Number of valence electrons of C = 4
• Number of valence electrons of O = 6
• So total number of valence electrons = [4+(3 × 6)] = 22
• Two extra electrons are also present
■ We will write the number as two items:
(a) Total number of ‘available valence electrons’ = 22
(b) Number of electrons to be added = 2
• Final number = [(a) ± (b)] = [(a) + (b)] = [22 + 2] = 24
Step 2: The skeletal structure
• The skeletal structure is shown in fig.4.52(a) below:
Step 3: Preliminary single bonds
• The four atoms are joined by '─' as shown in fig.4.52(b) above
Step 4: Preliminary distribution of electrons
(Remember that, only the 'available valence electrons' are distributed in this step)
• First make the three outer O atoms octet
♦ For that (3×8) = 24 electrons will be required
♦ But the number of 'available valence electrons' = 22
• So first, we will make the left and right O atoms octet
• Then give the remaining electrons to the top O atom
• This is shown in fig.c
• In the fig.c, we see that:
♦ The valence electrons of the O atoms are shown in green color
♦ The valence electrons of the C atom are shown in red color
• Left and right side O atoms have 8 electrons (including the one red dot in the single bonds)
♦ So the two O atoms use up (2 × 8) = 16 electrons
♦ The number of remaining electrons = (22-16) = 6
♦ These 6 electrons are given to the top O atom
• There are no more electrons to distribute
Step 5: Check for octet
• The left and right side O atoms have got 8 electrons each
• The top O atom has got only 6 electrons
♦ So this atom needs 2 more electrons
• The C atom has got only 6 electrons
♦ So this atom also needs 2 more electrons
■ Rearrangement: Change the preliminary single bond
♦ Change the top single bond to double bond as shown in the fig.d
• Two electrons from the top O is used for making the new bond
♦ Now the left and right side O atoms have octet
♦ The C atom also has octet
♦ But the top O atom has got only 6 electrons
• All the 22 electrons are used up. Still, complete octet is not achieved
• So, we will need external electrons
• Get two external electrons from any suitable source
• Give them to the top O atom
• This is shown in fig.e
• Now all atoms have octet
(v) But the two external electrons will create a charge of -2
• So we put the structure inside square brackets and put a -2 at the top right corner
• The structure in fig.4.52(e) is stable
2. Another possible rearrangement
• We already know how to obtain the structure in fig.4.52(e) above
♦ We obtained it by working from fig.4.52(b)
• That same fig.4.52(b) is shown again in fig.4.53(b) below:
• Earlier, we made the left and right O atoms octet
♦ This time, we make the top and right O atoms octet. This is shown in fig.4.53(c')
• Next we take two electrons from the left O atom and make a double bond
♦ This is shown in fig.4.53(d')
• Finally, we add the extra two electrons to the left side O atom to attain octet
♦ This is shown in fig.4.53(e')
• The structure in fig.4.53(e') is stable
3. Yet another possible rearrangement
• We already know how to obtain the structure in fig.4.52(e) above
♦ We obtained it by working from fig.4.52(b)
• That same fig.4.52(b) is shown again in fig.4.54(b) below:
• Earlier, we made the left and right O atoms octet
♦ This time, we make the top and left O atoms octet. This is shown in fig.4.54(c'')
• Next we take two electrons from the right O atom and make a double bond
♦ This is shown in fig.4.54(d'')
• Finally, we add the extra two electrons to the right side O atom to attain octet
♦ This is shown in fig.4.54(e'')
• The structure in fig.4.54(e'') is stable
4. So we have three possible structures of CO32-
♦ The structure in fig.4.52(e)
♦ The structure in fig.4.53(e')
♦ The structure in fig.4.54(e'')
• They are shown together in fig.4.55 below:
5. Now the next question arises:
■ In reality, which is the correct form in which CO32- exists? Fig.4.55 (e), (e') or (e'')?
• The answer can be written in 3 steps:
(i) A C-O single bond will have a length of 134 pm
(ii) A C=O double bond will have a length of 121 pm
(iii) With this information, we examine the bond lengths in an actual CO32- molecule
• Surprisingly, the actual values are different from 134 and 121
• In fact there are no 'values'. There is only one value. It is 128 pm
• The distance between C and O atoms in all the three pairs is 128 pm
6. The structures in figs (e) (e') and (e'') are called canonical structures of CO32-
• They are also called resonance structures of CO32-
7. Resonance structures are indicated by giving double headed arrows between them
(i) Sometimes, a single Lewis structure cannot describe a molecule accurately
• We may have to show two or more structure
(ii) Those structures will have similar energies
• Also positions of atoms will be similar in those structures
• But 'lone pairs' and 'bonds' will be different
• Those structures are called canonical structures or resonance structures
(iii) None of the resonance structures can be used to represent the actual structure
• The actual structure is more accurately described by a structure called hybrid of the resonance structures
• This structure is also called the resonance hybrid
1. Resonance stabilizes the molecule as the energy of the resonance hybrid is less than the energy of any single resonance structure
• This can be explained in 5 steps:
(i) Consider a resonance hybrid and it’s various resonance structures
(ii) Each of the resonance structures will have it’s own ‘quantity of energy’
(iii) The resonance hybrid will also have it’s own ‘quantity of energy’
(iv) The energy in (iii) will be less than any of the energies in (ii)
(v) So the 'phenomenon of resonance' helps the molecule to attain greater stability
2. Resonance averages the bond characteristics as a whole
• This can be explained in 6 steps:
(i) We have seen some of the bond characteristics:
Bond length, Bond angle, Bond enthalpy, Bond order
(ii) Take any one of them, say bond length
(iii) Each of the resonance structures will have 'it’s own bond lengths' for it’s various bonds
(iv) The resonance hybrid will also have 'it’s own bond lengths' for it’s various bonds
(v) The values in (iv) will be the averages of the corresponding values in (iii)
(vi) Here, we have considered bond length. The same can be written about other bond characteristics also
Example 1: Resonance structures of ozone
1. Let us first draw the Lewis dot structure of O3 (ozone) molecule
(We have seen the steps in an earlier section 4.2)
Step 1: Finding the number of dots
• Number of valence electrons of O = 6
• So total number of valence electrons = (3 × 6) = 18
Step 2: The skeletal structure
• The skeletal structure is shown in fig.4.44(a) below:
 |
| Fig.4.44 |
• The three atoms are joined by '─' as shown in fig.4.44(b) above
Step 4: Preliminary distribution of electrons
• First make the two outer O atoms octet
• Then give the remaining electrons to the central O atom
• This is shown in fig.c
• In the fig.c, we see that:
♦ The valence electrons of the outer O atoms are shown in green color
♦ The valence electrons of the central O atom are shown in red color
• Both the outer O atoms have 8 electrons (including the one red dot in the single bonds)
♦ So the number of electrons used up for making those O atoms octet = 16
♦ So the number of remaining electrons = (18-16) = 2
♦ These 2 electrons are given to the central O atom
• There are no more electrons to distribute
Step 5: Check for octet
• Both the outer O atoms have got 8 electrons each. They have attained octet
• The central O atom has got only 6 electrons. It has not attained octet
• Thus, the preliminary distribution needs to be changed
■ Rearrangement:
• In fig.c, take a lone pair from the left side O atom
• Using those two electrons, convert the left side single bond to a double bond
• This is shown in fig.d
• In fig.d, all the atoms have octet. So it is a stable O3 molecule
2. Another possible rearrangement
• We already know how to obtain the structure in fig.4.44(d) above
♦ We obtained it based on fig.4.44(c)
• That same fig.4.44(c) is shown again in fig.4.45(c) below:
 |
| Fig.4.45 |
• Using those two electrons, convert the right side single bond to a double bond
• This is shown in fig.4.45(d')
• In fig.d', all the atoms have octet. So it is also a stable O3 molecule
3. So we have two possible structures of O3
♦ One is the structure in fig.4.44(d)
♦ The other is the structure in fig.4.45(d')
• They are shown together in figs.4.46 below:
 |
| Fig.4.46 |
■ In reality, which is the correct form in which O3 exists? Fig.4.46 (d) or (d')?
• The answer can be written in 5 steps:
(i) An O-O single bond will have a length of 148 pm.
♦ This is shown in figs.4.47(a) and (b) below.
(ii) An O=O double bond will have a length of 121 pm
♦ This is shown in figs.4.47(a) and (b) below.
(iii) With this information, we examine an actual O3 molecule.
♦ We would expect the distance between one pair of O atoms to be 148 pm
♦ We would expect the distance between the other pair of O atoms to be 121 pm
(iv) But surprisingly, the actual values are different from both 148 and 121
• In fact there are no 'values'. There is only one value. It is 128 pm
• The distance between atoms in both pairs is 128 pm.
• This is shown in fig.4.47(c) below:
 |
| Fig.4.47 |
• (c) is different from both (a) and (b)
(v) Note the bonds in fig.c. They are neither single bonds nor double bonds
• This is indicated by the dashed lines
■ Fig.c represents the structure of O3 more accurately
5. The structures in (a) and (b) are called canonical structures
♦ They are also called resonance structures
(For some molecules, there will be more than two resonance structures)
6. Resonance structures are indicated by giving double headed arrows between them
• The structure in (c) is called the hybrid of the resonance structures
♦ It is also called the resonance hybrid
Example 2: Resonance structures of carbon dioxide
1. Let us first draw the Lewis dot structure of CO2 (carbon dioxide) molecule
(We have seen the steps in an earlier section 4.2)
Step 1: Finding the number of dots
• Number of valence electrons of C = 4
• Number of valence electrons of O = 6
• So total number of valence electrons = [4+(2 × 6)] = 16
Step 2: The skeletal structure
• The skeletal structure is shown in fig.4.48(a) below:
 |
| Fig.4.48 |
• The three atoms are joined by '─' as shown in fig.4.48(b) above
Step 4: Preliminary distribution of electrons
• First make the two outer O atoms octet
• Then give the remaining electrons to the central C atom
• This is shown in fig.c
• In the fig.c, we see that:
♦ The valence electrons of the outer O atoms are shown in green color
♦ The valence electrons of the central O atom are shown in red color
• Both the outer O atoms have 8 electrons (including the one red dot in the single bonds)
♦ So the number of electrons used up for making those O atoms octet = 16
♦ So the number of remaining electrons = (16-16) = 0
• There are no more electrons to distribute
Step 5: Check for octet
• Both the outer O atoms have got 8 electrons each. They have attained octet
• The central C atom has got only 4 electrons. It has not attained octet
• Thus, the preliminary distribution needs to be changed
■ Rearrangement:
• In fig.c, take a lone pair from the left side O atom
♦ Using those two electrons, convert the left side single bond to a double bond
• Again, in fig.c, take a lone pair from the right side O atom
♦ Using those two electrons, convert the right side single bond to a double bond
• This is shown in fig.d
• In fig.d, all the atoms have octet. So it is a stable CO2 molecule
2. Another possible rearrangement
• We already know how to obtain the structure in fig.4.48(d) above
♦ We obtained it based on fig.4.48(c)
• That same fig.4.48(c) is shown again in fig.4.49(c) below:
 |
| Fig.4.49 |
• Using those four electrons, convert the left side single bond to a triple bond
• This is shown in fig.4.49(d')
• In fig.4.49(d'), all the atoms have octet. So it is also a stable CO2 molecule
3. Yet another possible rearrangement
• The above rearrangement in fig.4.49(d') was based on fig.4.48(c)
• That same fig.4.48(c) is shown again in fig.4.50(c) below:
 |
| Fig.4.50 |
• Using those four electrons, convert the right side single bond to a triple bond
• This is shown in fig.4.50(d'')
• In fig.4.50(d''), all the atoms have octet. So it is also a stable CO2 molecule
4. So we have three possible structures of CO2
♦ The structure in fig.4.48(d)
♦ The structure in fig.4.49(d')
♦ The structure in fig.4.50(d'')
• They are shown together in fig.4.51 below:
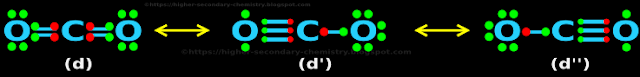 |
| Fig.4.51 |
■ In reality, which is the correct form in which CO2 exists? Fig.4.51 (d), (d') or (d'')?
• The answer can be written in 4 steps:
(i) A C-O single bond will have a length of 134 pm
(ii) A C=O double bond will have a length of 121 pm
(iii) A C≡O triple bond will have a length of 110 pm
(iv) With this information, we examine the bond lengths in an actual CO2 molecule
• Surprisingly, the actual values are different from 134, 121 and 110
• In fact there are no 'values'. There is only one value. It is 115 pm
• The distance between C and O atoms in both pairs is 115 pm
6. The structures in figs (d) (d') and (d'') are called canonical structures of CO2
• They are also called resonance structures of CO2
7. Resonance structures are indicated by giving double headed arrows between them
Example 3: Resonance structures of carbonate ion
(We have seen the structure in an earlier section 4.3. But there we did not explore the various possible arrangements)
Step 1: Finding the number of dots
• Number of valence electrons of C = 4
• Number of valence electrons of O = 6
• So total number of valence electrons = [4+(3 × 6)] = 22
• Two extra electrons are also present
■ We will write the number as two items:
(a) Total number of ‘available valence electrons’ = 22
(b) Number of electrons to be added = 2
• Final number = [(a) ± (b)] = [(a) + (b)] = [22 + 2] = 24
Step 2: The skeletal structure
• The skeletal structure is shown in fig.4.52(a) below:
 |
| Fig.4.52 |
• The four atoms are joined by '─' as shown in fig.4.52(b) above
Step 4: Preliminary distribution of electrons
(Remember that, only the 'available valence electrons' are distributed in this step)
• First make the three outer O atoms octet
♦ For that (3×8) = 24 electrons will be required
♦ But the number of 'available valence electrons' = 22
• So first, we will make the left and right O atoms octet
• Then give the remaining electrons to the top O atom
• This is shown in fig.c
• In the fig.c, we see that:
♦ The valence electrons of the O atoms are shown in green color
♦ The valence electrons of the C atom are shown in red color
• Left and right side O atoms have 8 electrons (including the one red dot in the single bonds)
♦ So the two O atoms use up (2 × 8) = 16 electrons
♦ The number of remaining electrons = (22-16) = 6
♦ These 6 electrons are given to the top O atom
• There are no more electrons to distribute
Step 5: Check for octet
• The left and right side O atoms have got 8 electrons each
• The top O atom has got only 6 electrons
♦ So this atom needs 2 more electrons
• The C atom has got only 6 electrons
♦ So this atom also needs 2 more electrons
■ Rearrangement: Change the preliminary single bond
♦ Change the top single bond to double bond as shown in the fig.d
• Two electrons from the top O is used for making the new bond
♦ Now the left and right side O atoms have octet
♦ The C atom also has octet
♦ But the top O atom has got only 6 electrons
• All the 22 electrons are used up. Still, complete octet is not achieved
• So, we will need external electrons
• Get two external electrons from any suitable source
• Give them to the top O atom
• This is shown in fig.e
• Now all atoms have octet
(v) But the two external electrons will create a charge of -2
• So we put the structure inside square brackets and put a -2 at the top right corner
• The structure in fig.4.52(e) is stable
2. Another possible rearrangement
• We already know how to obtain the structure in fig.4.52(e) above
♦ We obtained it by working from fig.4.52(b)
• That same fig.4.52(b) is shown again in fig.4.53(b) below:
 |
| Fig.4.53 |
♦ This time, we make the top and right O atoms octet. This is shown in fig.4.53(c')
• Next we take two electrons from the left O atom and make a double bond
♦ This is shown in fig.4.53(d')
• Finally, we add the extra two electrons to the left side O atom to attain octet
♦ This is shown in fig.4.53(e')
• The structure in fig.4.53(e') is stable
3. Yet another possible rearrangement
• We already know how to obtain the structure in fig.4.52(e) above
♦ We obtained it by working from fig.4.52(b)
• That same fig.4.52(b) is shown again in fig.4.54(b) below:
 |
| Fig.4.54 |
♦ This time, we make the top and left O atoms octet. This is shown in fig.4.54(c'')
• Next we take two electrons from the right O atom and make a double bond
♦ This is shown in fig.4.54(d'')
• Finally, we add the extra two electrons to the right side O atom to attain octet
♦ This is shown in fig.4.54(e'')
• The structure in fig.4.54(e'') is stable
4. So we have three possible structures of CO32-
♦ The structure in fig.4.52(e)
♦ The structure in fig.4.53(e')
♦ The structure in fig.4.54(e'')
• They are shown together in fig.4.55 below:
 |
| Fig.4.55 |
■ In reality, which is the correct form in which CO32- exists? Fig.4.55 (e), (e') or (e'')?
• The answer can be written in 3 steps:
(i) A C-O single bond will have a length of 134 pm
(ii) A C=O double bond will have a length of 121 pm
(iii) With this information, we examine the bond lengths in an actual CO32- molecule
• Surprisingly, the actual values are different from 134 and 121
• In fact there are no 'values'. There is only one value. It is 128 pm
• The distance between C and O atoms in all the three pairs is 128 pm
6. The structures in figs (e) (e') and (e'') are called canonical structures of CO32-
• They are also called resonance structures of CO32-
7. Resonance structures are indicated by giving double headed arrows between them
• We will practice using 2 more examples: SO3 and NO3-
♦ The steps can be seen here
♦ The steps can be seen here
■ Now we can write the definition of resonance structures. It can be written in 3 steps:
• We may have to show two or more structure
(ii) Those structures will have similar energies
• Also positions of atoms will be similar in those structures
• But 'lone pairs' and 'bonds' will be different
• Those structures are called canonical structures or resonance structures
(iii) None of the resonance structures can be used to represent the actual structure
• The actual structure is more accurately described by a structure called hybrid of the resonance structures
• This structure is also called the resonance hybrid
■ We must always remember two important points related to resonance:
• This can be explained in 5 steps:
(i) Consider a resonance hybrid and it’s various resonance structures
(ii) Each of the resonance structures will have it’s own ‘quantity of energy’
(iii) The resonance hybrid will also have it’s own ‘quantity of energy’
(iv) The energy in (iii) will be less than any of the energies in (ii)
(v) So the 'phenomenon of resonance' helps the molecule to attain greater stability
2. Resonance averages the bond characteristics as a whole
• This can be explained in 6 steps:
(i) We have seen some of the bond characteristics:
Bond length, Bond angle, Bond enthalpy, Bond order
(ii) Take any one of them, say bond length
(iii) Each of the resonance structures will have 'it’s own bond lengths' for it’s various bonds
(iv) The resonance hybrid will also have 'it’s own bond lengths' for it’s various bonds
(v) The values in (iv) will be the averages of the corresponding values in (iii)
(vi) Here, we have considered bond length. The same can be written about other bond characteristics also
Many misconceptions are associated with resonance. We will analyze four misconceptions and get to know the facts
1. The existence of resonance structures in a sample
• This can be analysed in 3 steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) Take any sample of ozone
• The fact is that, we will never find any of those two resonance structures in any ozone sample
(iii) The resonance structures exist only in drawings. The true structure is the hybrid of those resonance structures
2. The existence of resonance structures based on time
• This can be analysed in 3 steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) Take any sample of ozone
• There is a popular belief:
♦ At some instances of time, the sample will contain the O3 molecules in one canonical form
♦ At some other instances of time, the sample will contain the O3 molecules in the other canonical form
(iii) This is totally wrong
• At any instant that we take, there will be only one form, which is the hybrid
3. Equilibrium between various canonical forms
• This can be analysed in 3 steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) Take any sample of ozone
• There was a popular belief:
♦ The sample contains both the canonical forms in equal quantities
♦ If one canonical form is in excess quantity, it will be gradually converted into the other form
✰ This conversion will continue until both forms are in equal quantities
(iii) This is totally wrong
• The canonical forms do not even exist. So there is no question of attaining an equilibrium between them
4. Representation of the molecule
• This can be analysed in steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) One may think that he/she can represent the O3 by drawing 'any one' of it's Lewis structures
(iii) But the fact is this:
• If we draw just any one, we will be conveying the 'wrong information' that, one bond in O3 is a single bond and the other is a double bond
(iii) It is impossible to represent such molecules by a single Lewis dot structure
• So it is compulsory to draw all the canonical structures and show double headed arrows between them
Solved example 4.5
H3PO3 can be represented by structures (a) and (b) shown in fig.4.64 below. Can these two structures be taken as the canonical forms of the resonance hybrid representing H3PO3 ?
If not, give reasons for the same.
Solution:
In the two given structures, the positions of atoms are not the same. So they are not the canonical forms of the resonance hybrid representing H3PO3
1. The existence of resonance structures in a sample
• This can be analysed in 3 steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) Take any sample of ozone
• The fact is that, we will never find any of those two resonance structures in any ozone sample
(iii) The resonance structures exist only in drawings. The true structure is the hybrid of those resonance structures
2. The existence of resonance structures based on time
• This can be analysed in 3 steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) Take any sample of ozone
• There is a popular belief:
♦ At some instances of time, the sample will contain the O3 molecules in one canonical form
♦ At some other instances of time, the sample will contain the O3 molecules in the other canonical form
(iii) This is totally wrong
• At any instant that we take, there will be only one form, which is the hybrid
3. Equilibrium between various canonical forms
• This can be analysed in 3 steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) Take any sample of ozone
• There was a popular belief:
♦ The sample contains both the canonical forms in equal quantities
♦ If one canonical form is in excess quantity, it will be gradually converted into the other form
✰ This conversion will continue until both forms are in equal quantities
(iii) This is totally wrong
• The canonical forms do not even exist. So there is no question of attaining an equilibrium between them
4. Representation of the molecule
• This can be analysed in steps:
(i) Let us take the example of ozone
♦ We saw that there are 2 resonance structures for ozone
(ii) One may think that he/she can represent the O3 by drawing 'any one' of it's Lewis structures
(iii) But the fact is this:
• If we draw just any one, we will be conveying the 'wrong information' that, one bond in O3 is a single bond and the other is a double bond
(iii) It is impossible to represent such molecules by a single Lewis dot structure
• So it is compulsory to draw all the canonical structures and show double headed arrows between them
Now we will see a solved example
H3PO3 can be represented by structures (a) and (b) shown in fig.4.64 below. Can these two structures be taken as the canonical forms of the resonance hybrid representing H3PO3 ?
 |
| Fig.4.64 |
Solution:
In the two given structures, the positions of atoms are not the same. So they are not the canonical forms of the resonance hybrid representing H3PO3
In the next section, we will see polarity of bonds
No comments:
Post a Comment