In the previous section, we saw the basics about resonance structures. In this section, we will see polarity of bonds
1. We have seen two types of bonds:
♦ Ionic bonds
✰ This involves transfer of electrons
♦ Covalent bonds
✰ This involves sharing of electrons
2. Consider ionic bonds
• In some ionic bonds, the cation is able to pull the ‘extra electrons of the anion’
♦ Then, the ‘extra electrons of the anion’ will no longer belong to the anion alone
♦ They will belong to both the anion and the cation
• Thus a little 'covalent character' is induced in that ionic bond
■ An ionic bond with no covalent characteristics is called an ‘ideal ionic bond’
3. Consider covalent bonds
• In some covalent bonds, the ‘more electronegative atom’ is able to pull the ‘shared pairs of electrons’
♦ Then, that ‘more electronegative atom’ acquires a 'partial negative charge'
♦ Also, the 'less electronegative atom' acquires a 'partial positive charge'
• Thus a little 'ionic character' is induced in that covalent bond
■ A covalent bond with no ionic characteristics is called an ‘ideal covalent bond’
4. In reality,
• No ionic bond is an ideal ionic bond
♦ All ionic bonds will have some covalent character
• No covalent bond is an ideal covalent bond
♦ All covalent bonds will have some ionic character
1. Consider the homonuclear diatomic molecules like H2, O2, Cl2, N2, F2 etc.,
• In those molecules, the shared pairs of electrons are ‘attracted equally’ by the two atoms
♦ So the shared pairs will be exactly midway between the two nuclei
■ In such molecules, the covalent bonds are called non-polar covalent bonds
2. Consider heteronuclear diatomic molecules like HCl, HF etc.,
• Let us compare H with Cl and F
♦ Cl and F are more electronegative
• So in those molecules, the shared pair gets displaced more towards the more electronegative atom
■ In such molecules, the covalent bonds are called polar covalent bonds
We will see a solved example
Solved example 4.6
Arrange the bonds in order of increasing ionic character in the molecules: LiF, K2O, N2, SO2 and ClF3
Solution:
1. Consider LiF
(i) Electronegativity values:
Li - 1.0, F - 4.0
(ii) Difference in electronegativity values = (4.0 - 1.0) = 3.0
2. Consider K2O
(i) Electronegativity values:
K - 0.8, O - 3.5
(ii) Difference in electronegativity values = (3.5 - 0.8) = 2.7
3. Consider N2
(i) Electronegativity values:
N - 3.0
(ii) Difference in electronegativity values = (3.0 - 3.0) = 0.0
4. Consider SO2
(i) Electronegativity values:
S - 2.5, O - 3.5
(ii) Difference in electronegativity values = (3.5 - 2.5) = 1.0
5. Consider ClF3
(i) Electronegativity values:
Cl - 3.0, F - 4.0
(ii) Difference in electronegativity values = (4.0 - 3.0) = 1.0
6. Arranging the differences in increasing order, we get:
0.0 < [1.0 = 1.0] < 2.7 < 3.0
7. Greater the difference, greater is the ionic character. So we get:
N2 < [SO2 = ClF3] < K2O < LiF
8. Consider [SO2 = ClF3]
• The F is more electronegative than O
♦ Also, in SO2, two O atoms are pulling onto S
♦ But in ClF3, three F atoms are pulling onto Cl
• So ClF3 is more ionic
• Thus the correct order is:
N2 < SO2 < ClF3 < K2O < LiF
• The more electronegative atom acquires a partial negative charge indicated by Q-
• The less electronegative atom acquires a partial positive charge indicated by Q+
• Magnitudes of both charges will be equal to 'Q'
♦ But their signs will be opposite
2. So in a molecule with polar covalent bond, we have:
♦ A positive charge Q+ at one end of the molecule
✰ This is the end where the less electronegative atom is situated
♦ A negative charge Q- at the other end of the molecule
✰ This is the end where the more electronegative atom is situated
3. Note down the ‘distance between the centers of the two charges’
• Let this distance be 'r'
■ Then the product (Q × r) is called the dipole moment possessed by the molecule
4. Dipole moment is denoted by the Greek letter '𝝁'
• So mathematically, we can write: 𝝁 = Q r
♦ The charge Q is measured in coulombs (C)
♦ The distance r is measured in meters (m)
♦ So the unit of 𝝁 will be coulomb meter (C m)
5. But When expressed in C m, the dipole moment will be very small
(With a lot of zeros after the decimal point)
• So we usually use the unit Debye
♦ It’s symbol is D
• 1 D is equal to 3.33564 × 10-30 C m
6. Dipole moment is a vector quantity
• So it has both magnitude and direction
7. Whenever two opposite charges are placed at a distance apart, we can calculate the dipole moment
• In physics classes, we come across this situation on many occasions
• There, we denote the dipole moment using a small arrow
♦ The tail of the arrow is at the center of the negative charge
♦ The head points towards the center of the positive charge
8. But in chemistry, we follow a different notation
• Instead of the arrow, we use a crossed arrow
♦ This crossed arrow is put on the Lewis structure of the molecule
✰ The cross is at the positive end
✰ The arrow head points towards the negative end
9. Using this notation, the dipole moment of HF is shown in fig.4.65 below:
• The crossed arrow is shown in yellow color
• The crossed arrow symbolizes 'a direction'
♦ It is the direction in which the electrons shift
• In our present case, the electron shifts from H to the 'more electronegative F'
10. HF is a diatomic molecule. Next we will see some polyatomic molecules
• Consider the water molecule
• Though we sometimes represent the water molecule as H-O-H, the two O-H bonds do not lie on the same line
♦ If the two bonds lie on the same line, the angle between them would be 180o
♦ This is shown in fig.4.66(b) below:
• But the actual angle is 104.5o
♦ This is shown in fig.4.66(c) above
11. We will have two dipole moments
♦ The dipole moment between the 'left H' and O
♦ The dipole moment between the 'right H' and O
• They are shown in yellow color in fig.4.66(d) above
• The dipole moments are due to the 'pulling of electrons' by the O atom
12. But we want the dipole moment of the ‘molecule as a whole’
■ For that, we calculate the vector sum
• We have seen that, dipole moments are vector quantities
♦ So they can be added using 'principles of vector addition'
✰ Details can be seen here
13. Let us add the two dipole moment vectors:
(i) Fig.4.67(a) below shows the left side dipole moment
• It is resolved into rectangular components
♦ The horizontal component is shown in red color
♦ The vertical component is shown in green color
(ii) For resolving the vector into it's rectangular components, we need the value of 𝞱 shown in fig.4.67(a)
• It can be easily calculated as shown in fig.4.67(b)
• All we need to do is: Draw a right triangle indicated by the magenta dashed lines
(iii) Once we know 𝞱, the rectangular components can be calculated as follows:
♦ The horizontal component (red) in fig.6.67(a) is calculated using the cosine of 𝞱
♦ The vertical component (green) in fig.6.67(a) is calculated using the sine of 𝞱
14. Next we repeat the same procedure for the right dipole moment
• That is.,
♦ We find the horizontal component (red) in fig.6.67(c)
♦ We find the vertical component (green) in fig.6.67(c)
15. Now we can do the vector addition
• We add the similar components:
♦ We add the reds in fig.a and fig.b
♦ We add the greens in fig.a and fig.b
16. The two dipole moments in the earlier fig.6.66(d) are equal in magnitude and have the same 𝞱
• So the two reds will have the same magnitudes
• The two greens will also have the same magnitude
17. While adding, we see that:
• The two reds have opposite directions
♦ So they will cancel each other
■ So the final resultant will not have a horizontal component
• The two greens have the same direction
♦ So they will add up
18. Since the reds cancel each other, we need to consider the greens only
• The resultant obtained from the two greens is shown in fig.6.67(d)
♦ It is shown as a thick green crossed arrow
♦ It is the resultant dipole moment
19. Scientists have determined this resultant
• It's magnitude is: 1.85 D
1. Here, the two Be-F bonds lie on the same line
• The angle between the two bonds is 180o
• This is shown in fig.6.68(b) below:
2. We will have two dipole moments
♦ The dipole moment between the 'left F' and Be
♦ The dipole moment between the 'right F' and Be
• They are shown in yellow color in fig.4.68(c) above
• The dipole moments are due to the 'pulling of electrons' by the F atoms
3. But we want the dipole moment of the ‘molecule as a whole’
■ For that, we calculate the vector sum
• We have seen that, dipole moments are vector quantities
♦ So they can be added using 'principles of vector addition'
✰ Details can be seen here
4. Let us add the two dipole moment vectors:
(i) We see that, both the vectors are horizontal
♦ So there is no need to resolve them into rectangular components
(ii) The two vectors are equal in magnitude. But they have opposite directions
♦ So they will cancel each other
(iii) So the resultant will be a null vector
• It is shown in fig.d
• A null vector has zero magnitude and hence no direction
5. We can write:
The dipole moment of BeF2 is zero
1. In this case, there are three bonds
♦ All of them are B-F bonds
♦ Those bonds do not lie on the same line
2. The orientations of the bonds can be described in two steps:
(i) From among the three bonds, take any two
(ii) The angle between those two bonds will be 120o
3. This is a simple orientation
• The '120o' indicates that, the bonds are distributed uniformly around the central B atom
• This is shown in fig.4.69(b)
[Remember that (3 × 120) = 360. The angle of a full circel is 360o]
4. We will have three dipole moments
♦ The dipole moment between the 'left F' and B
♦ The dipole moment between the 'top F' and B
♦ The dipole moment between the 'bottom F' and B
• They are shown in yellow color in fig.4.69(c) above
• The dipole moments are due to the 'pulling of electrons' by the F atoms
5. But we want the dipole moment of the ‘molecule as a whole’
■ For that, we calculate the vector sum
• We have seen that, dipole moments are vector quantities
♦ So they can be added using 'principles of vector addition'
✰ Details can be seen here
6. Let us add the three dipole moment vectors:
• This is a simple case of vector addition. We need not show the detailed steps. It is a 'mental math' problem
• The results of addition can be summarised in 7 steps:
(i) The leftside vector is horizontal. So there is no need to resolve it into rectangular components
(ii) The top vector is inclined. So it can be resolved
♦ Let us call the horizontal component as 'red'
♦ Let us call the vertical component as 'green'
(iii) The bottom vector is inclined. So it can be resolved
♦ Let us call the horizontal component as 'red'
♦ Let us call the vertical component as 'green'
(iv) The greens in (ii) and (ii) are equal and opposite
♦ So they cancel each other
■ The final resultant will not have any vertical component
(v) The reds in (ii) and (iii) are equal. Also, they act in the same direction
• So they add up
(vi) Consider the two vectors:
♦ The resultant vector obtained in (v)
♦ The vector mentioned in (i)
• These two vectors are equal and opposite
• So the resultant of these two vectors is a null vector
(viii) Thus the final resultant of all the three vectors is a null vector
• It is shown in fig.4.69(d)
• A null vector has zero magnitude and hence no direction
7. We can write:
• The dipole moment of BF3 is zero
8. This type of vector addition problems are encountered frequently in physics classes
• If the reader has any doubt, he/she may draw the necessary diagrams like those we saw in fig.6.68 earlier
• It is important to become convinced that, all steps written in (6) are valid
• OR, the reader may use any alternate steps to prove that the resultant is null vector
1. We have seen two types of bonds:
♦ Ionic bonds
✰ This involves transfer of electrons
♦ Covalent bonds
✰ This involves sharing of electrons
2. Consider ionic bonds
• In some ionic bonds, the cation is able to pull the ‘extra electrons of the anion’
♦ Then, the ‘extra electrons of the anion’ will no longer belong to the anion alone
♦ They will belong to both the anion and the cation
• Thus a little 'covalent character' is induced in that ionic bond
■ An ionic bond with no covalent characteristics is called an ‘ideal ionic bond’
3. Consider covalent bonds
• In some covalent bonds, the ‘more electronegative atom’ is able to pull the ‘shared pairs of electrons’
♦ Then, that ‘more electronegative atom’ acquires a 'partial negative charge'
♦ Also, the 'less electronegative atom' acquires a 'partial positive charge'
• Thus a little 'ionic character' is induced in that covalent bond
■ A covalent bond with no ionic characteristics is called an ‘ideal covalent bond’
4. In reality,
• No ionic bond is an ideal ionic bond
♦ All ionic bonds will have some covalent character
• No covalent bond is an ideal covalent bond
♦ All covalent bonds will have some ionic character
Polarity in covalent bonds
• In those molecules, the shared pairs of electrons are ‘attracted equally’ by the two atoms
♦ So the shared pairs will be exactly midway between the two nuclei
■ In such molecules, the covalent bonds are called non-polar covalent bonds
2. Consider heteronuclear diatomic molecules like HCl, HF etc.,
• Let us compare H with Cl and F
♦ Cl and F are more electronegative
• So in those molecules, the shared pair gets displaced more towards the more electronegative atom
■ In such molecules, the covalent bonds are called polar covalent bonds
We will see a solved example
Solved example 4.6
Arrange the bonds in order of increasing ionic character in the molecules: LiF, K2O, N2, SO2 and ClF3
Solution:
1. Consider LiF
(i) Electronegativity values:
Li - 1.0, F - 4.0
(ii) Difference in electronegativity values = (4.0 - 1.0) = 3.0
2. Consider K2O
(i) Electronegativity values:
K - 0.8, O - 3.5
(ii) Difference in electronegativity values = (3.5 - 0.8) = 2.7
3. Consider N2
(i) Electronegativity values:
N - 3.0
(ii) Difference in electronegativity values = (3.0 - 3.0) = 0.0
4. Consider SO2
(i) Electronegativity values:
S - 2.5, O - 3.5
(ii) Difference in electronegativity values = (3.5 - 2.5) = 1.0
5. Consider ClF3
(i) Electronegativity values:
Cl - 3.0, F - 4.0
(ii) Difference in electronegativity values = (4.0 - 3.0) = 1.0
6. Arranging the differences in increasing order, we get:
0.0 < [1.0 = 1.0] < 2.7 < 3.0
7. Greater the difference, greater is the ionic character. So we get:
N2 < [SO2 = ClF3] < K2O < LiF
8. Consider [SO2 = ClF3]
• The F is more electronegative than O
♦ Also, in SO2, two O atoms are pulling onto S
♦ But in ClF3, three F atoms are pulling onto Cl
• So ClF3 is more ionic
• Thus the correct order is:
N2 < SO2 < ClF3 < K2O < LiF
Dipole moment
1. Consider a polar covalent bond• The less electronegative atom acquires a partial positive charge indicated by Q+
• Magnitudes of both charges will be equal to 'Q'
♦ But their signs will be opposite
2. So in a molecule with polar covalent bond, we have:
♦ A positive charge Q+ at one end of the molecule
✰ This is the end where the less electronegative atom is situated
♦ A negative charge Q- at the other end of the molecule
✰ This is the end where the more electronegative atom is situated
3. Note down the ‘distance between the centers of the two charges’
• Let this distance be 'r'
■ Then the product (Q × r) is called the dipole moment possessed by the molecule
4. Dipole moment is denoted by the Greek letter '𝝁'
• So mathematically, we can write: 𝝁 = Q r
♦ The charge Q is measured in coulombs (C)
♦ The distance r is measured in meters (m)
♦ So the unit of 𝝁 will be coulomb meter (C m)
5. But When expressed in C m, the dipole moment will be very small
(With a lot of zeros after the decimal point)
• So we usually use the unit Debye
♦ It’s symbol is D
• 1 D is equal to 3.33564 × 10-30 C m
6. Dipole moment is a vector quantity
• So it has both magnitude and direction
7. Whenever two opposite charges are placed at a distance apart, we can calculate the dipole moment
• In physics classes, we come across this situation on many occasions
• There, we denote the dipole moment using a small arrow
♦ The tail of the arrow is at the center of the negative charge
♦ The head points towards the center of the positive charge
8. But in chemistry, we follow a different notation
• Instead of the arrow, we use a crossed arrow
♦ This crossed arrow is put on the Lewis structure of the molecule
✰ The cross is at the positive end
✰ The arrow head points towards the negative end
9. Using this notation, the dipole moment of HF is shown in fig.4.65 below:
 |
| Fig.4.65 |
• The crossed arrow symbolizes 'a direction'
♦ It is the direction in which the electrons shift
• In our present case, the electron shifts from H to the 'more electronegative F'
10. HF is a diatomic molecule. Next we will see some polyatomic molecules
• Consider the water molecule
• Though we sometimes represent the water molecule as H-O-H, the two O-H bonds do not lie on the same line
♦ If the two bonds lie on the same line, the angle between them would be 180o
♦ This is shown in fig.4.66(b) below:
 |
| Fig.6.66 |
♦ This is shown in fig.4.66(c) above
11. We will have two dipole moments
♦ The dipole moment between the 'left H' and O
♦ The dipole moment between the 'right H' and O
• They are shown in yellow color in fig.4.66(d) above
• The dipole moments are due to the 'pulling of electrons' by the O atom
12. But we want the dipole moment of the ‘molecule as a whole’
■ For that, we calculate the vector sum
• We have seen that, dipole moments are vector quantities
♦ So they can be added using 'principles of vector addition'
✰ Details can be seen here
13. Let us add the two dipole moment vectors:
(i) Fig.4.67(a) below shows the left side dipole moment
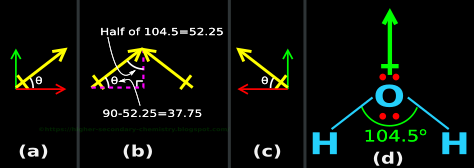 |
| Fig.6.67 |
♦ The horizontal component is shown in red color
♦ The vertical component is shown in green color
(ii) For resolving the vector into it's rectangular components, we need the value of 𝞱 shown in fig.4.67(a)
• It can be easily calculated as shown in fig.4.67(b)
• All we need to do is: Draw a right triangle indicated by the magenta dashed lines
(iii) Once we know 𝞱, the rectangular components can be calculated as follows:
♦ The horizontal component (red) in fig.6.67(a) is calculated using the cosine of 𝞱
♦ The vertical component (green) in fig.6.67(a) is calculated using the sine of 𝞱
14. Next we repeat the same procedure for the right dipole moment
• That is.,
♦ We find the horizontal component (red) in fig.6.67(c)
♦ We find the vertical component (green) in fig.6.67(c)
15. Now we can do the vector addition
• We add the similar components:
♦ We add the reds in fig.a and fig.b
♦ We add the greens in fig.a and fig.b
16. The two dipole moments in the earlier fig.6.66(d) are equal in magnitude and have the same 𝞱
• So the two reds will have the same magnitudes
• The two greens will also have the same magnitude
17. While adding, we see that:
• The two reds have opposite directions
♦ So they will cancel each other
■ So the final resultant will not have a horizontal component
• The two greens have the same direction
♦ So they will add up
18. Since the reds cancel each other, we need to consider the greens only
• The resultant obtained from the two greens is shown in fig.6.67(d)
♦ It is shown as a thick green crossed arrow
♦ It is the resultant dipole moment
19. Scientists have determined this resultant
• It's magnitude is: 1.85 D
Next, we will see the dipole moment in another polyatomic molecule: BeF2
• The angle between the two bonds is 180o
• This is shown in fig.6.68(b) below:
 |
| Fig.4.68 |
♦ The dipole moment between the 'left F' and Be
♦ The dipole moment between the 'right F' and Be
• They are shown in yellow color in fig.4.68(c) above
• The dipole moments are due to the 'pulling of electrons' by the F atoms
3. But we want the dipole moment of the ‘molecule as a whole’
■ For that, we calculate the vector sum
• We have seen that, dipole moments are vector quantities
♦ So they can be added using 'principles of vector addition'
✰ Details can be seen here
4. Let us add the two dipole moment vectors:
(i) We see that, both the vectors are horizontal
♦ So there is no need to resolve them into rectangular components
(ii) The two vectors are equal in magnitude. But they have opposite directions
♦ So they will cancel each other
(iii) So the resultant will be a null vector
• It is shown in fig.d
• A null vector has zero magnitude and hence no direction
5. We can write:
The dipole moment of BeF2 is zero
Next, we will see the dipole moment in another polyatomic molecule: BF3
♦ All of them are B-F bonds
♦ Those bonds do not lie on the same line
2. The orientations of the bonds can be described in two steps:
(i) From among the three bonds, take any two
(ii) The angle between those two bonds will be 120o
3. This is a simple orientation
• The '120o' indicates that, the bonds are distributed uniformly around the central B atom
• This is shown in fig.4.69(b)
[Remember that (3 × 120) = 360. The angle of a full circel is 360o]
 |
| Fig.4.69 |
♦ The dipole moment between the 'left F' and B
♦ The dipole moment between the 'top F' and B
♦ The dipole moment between the 'bottom F' and B
• They are shown in yellow color in fig.4.69(c) above
• The dipole moments are due to the 'pulling of electrons' by the F atoms
5. But we want the dipole moment of the ‘molecule as a whole’
■ For that, we calculate the vector sum
• We have seen that, dipole moments are vector quantities
♦ So they can be added using 'principles of vector addition'
✰ Details can be seen here
6. Let us add the three dipole moment vectors:
• This is a simple case of vector addition. We need not show the detailed steps. It is a 'mental math' problem
• The results of addition can be summarised in 7 steps:
(i) The leftside vector is horizontal. So there is no need to resolve it into rectangular components
(ii) The top vector is inclined. So it can be resolved
♦ Let us call the horizontal component as 'red'
♦ Let us call the vertical component as 'green'
(iii) The bottom vector is inclined. So it can be resolved
♦ Let us call the horizontal component as 'red'
♦ Let us call the vertical component as 'green'
(iv) The greens in (ii) and (ii) are equal and opposite
♦ So they cancel each other
■ The final resultant will not have any vertical component
(v) The reds in (ii) and (iii) are equal. Also, they act in the same direction
• So they add up
(vi) Consider the two vectors:
♦ The resultant vector obtained in (v)
♦ The vector mentioned in (i)
• These two vectors are equal and opposite
• So the resultant of these two vectors is a null vector
(viii) Thus the final resultant of all the three vectors is a null vector
• It is shown in fig.4.69(d)
• A null vector has zero magnitude and hence no direction
7. We can write:
• The dipole moment of BF3 is zero
8. This type of vector addition problems are encountered frequently in physics classes
• If the reader has any doubt, he/she may draw the necessary diagrams like those we saw in fig.6.68 earlier
• It is important to become convinced that, all steps written in (6) are valid
• OR, the reader may use any alternate steps to prove that the resultant is null vector
In the next section, we will see the dipole moment of ammonia molecule
No comments:
Post a Comment