In the previous section, we completed a discussion on chemical bonding and molecular structure. In this chapter, we will see states of matter
• So our next aim is to learn about 'attractive forces between molecules'
• There are four types of attractive forces:
(i) London force (ii) Dipole-dipole force (iii) Dipole-induced dipole force (iv) Hydrogen bond
• First we will see London force. The details can be written in 12 steps:
1. We know that in an atom, the electrons are arranged symmetrically around the nucleus
• So we will see a ‘spherical electron cloud’ around the nucleus
2. Two such atoms are shown in fig.5.1(a) below
♦ They are named as: Atom A and Atom B
♦ They are situated in the close vicinity of each other
 |
| Fig.5.1 |
3. Sometimes, the electron cloud in Atom A becomes unsymmetrical
• This is shown in fig.b
• The electron cloud has become denser on the right side
• As a result, the right side gets a partial negative charge (δ-) and the left side gets a partial positive charge (δ+)
■ We say that: A dipole is developed in Atom A
4. The effect of this dipole will be felt in the Atom B, which is close by
• The electron cloud in the left side of B will be repelled away to the right side
• As a result, the right side gets a partial negative charge (δ-) and the left side gets a partial positive charge (δ+)
• This is also shown in fig.b
■ We say that: A dipole is developed in Atom B also
5. Thus a electrostatic force of attraction comes into effect between A and B
• This is indicated by the dashed line in fig.c
• So atoms A and B will tend to remain together as a single group
6. If there was no 'distortion in the cloud of A', none of this would have happened
• The two atoms A and B would have remained independent of each other
■ We can say: A 'distortion in the cloud of A' caused A and B to attract each other
7. Now another question arises:
■ How long will the attraction last?
• The answer can be written in 4 steps:
(i) The attraction will last as long as the cloud in A remains distorted
(ii) But unfortunately, the distortion will last only for a very short duration
♦ It is an instantaneous distortion
(iii) So the attraction between the two dipoles is also instantaneous
(iv) After that instantaneous distortion, the two clouds will return back to their original shapes and the attraction will cease to exist
8. In the fig.5.1, we have shown two atoms
• But this type of instantaneous attraction can occur between molecules also
9. This type of attraction was discovered by the German scientist Fritz London
■ So the force of attraction between two temporary dipoles is called London force
■ Another name for London force is: dispersion force
10. The magnitude of this force decreases with the increase in distance 'r' between the particles
11. When two charged particles interact (attraction or repulsion), a potential energy is produced
• This is similar to:
♦ The potential energy of a stone kept at a height above the surface of the earth
✰ The potential energy is created due to the attraction between earth and the stone
• We will learn about 'potential energy in the case of charged particles' in higher classes
12. In our present case, the energy will be proportional to $\mathbf\small{\rm{\frac{1}{r^6}}}$
• So we can write:
♦ The energy is inversely proportional to the sixth power of the distance between the two particles
13. London forces are important only at short distances (~ 500 pm)
14. Magnitude of the London force depend on the polarisability of the particles
♦ If the particles are highly polarisable, we get a strong London force
♦ If the particles are only feebly polarisable, we get only a feeble London force
• Next we will see dipole-dipole force. Details can be written in 5 steps
1. We have seen the details about polar molecules in section 4.10
• Consider an HCl molecule
♦ The electron cloud in HCl suffers a permanent distortion towards the Cl end
♦ This is shown in the fig.5.2(a) below:
 |
| Fig.5.2 |
• As a result of this distortion, the 'Cl end' gets a partial negative charge (δ-) and the 'H end' gets a partial positive charge (δ+)
• This is indicated by the dashed line in fig.5.2(b)
■ This attraction is called dipole-dipole force
3. The magnitude of this force decreases with the increase in distance 'r' between the particles
4. As in the case of London force, in dipole-dipole force also, there is potential energy
• If the sample is a solid, all the dipoles will be stationary
♦ Then, the energy will be proportional to $\mathbf\small{\rm{\frac{1}{r^3}}}$
• If the sample is given external energy, the dipoles will start to rotate about a suitable axis
♦ In such a situation, the energy will be proportional to $\mathbf\small{\rm{\frac{1}{r^6}}}$
5. Consider a sample of polar molecules
• We saw that, dipole-dipole force will be present between the molecules in that sample
• In addition to that dipole-dipole forces, London forces will also be acting
• So there is a net increase in the ‘interaction between particles’ in a sample of polar molecules
Next we will see dipole-induced dipole force. Details can be written in 8 steps
1. In fig.5.3(a) below, a non-polar molecule is placed in the close vicinity of a polar molecule
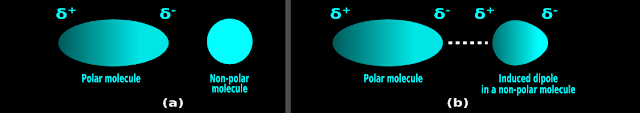 |
| Fig.5.3 |
• There is a denser electron cloud on the right side of the polar molecule
2. This denser electron cloud will repel the electron cloud of the near by non-polar molecule
• Thus the electron cloud of the non-polar molecule will become denser on the right side
3. So the right side gets a partial negative charge (δ-) and the left side gets a partial positive charge (δ+)
■ Thus a dipole is induced in the non-polar molecule
4. Now the two molecules will attract each other. This is indicated by the dashed line in fig.b
This attractive force is called dipole-induced dipole force
5. The magnitude of this force decreases with the increase in distance 'r' between the particles
6. As in the case of London force and dipole-dipole force, in dipole-induced dipole force also, there is potential energy
♦ This energy is proportional to $\mathbf\small{\rm{\frac{1}{r^6}}}$
7. Magnitude of the dipole-induced dipole force depends on two factors:
(i) Magnitude of the permanent dipole of the polar molecule
(ii) Polarisability of the non-polar molecule
• We have seen in a previous section that, if a molecule or atom is large in size, it’s polarisability will be high
8. Consider a sample containing both polar molecules and non-polar molecules
• We saw that, dipole-induced dipole force will be present between the molecules in that sample
• In addition to that dipole-dipole forces, London forces will also be acting
• So there is a net increase in the ‘interaction between particles’ in that sample
Next we will see hydrogen bond. Details can be written in 2 steps
1. We have already seen the formation of hydrogen bond in section 4.40
• We can write:
In a sample of NH, OH or HF, the molecules will be attracting each other by hydrogen bonds
2. Energy of hydrogen bond varies from 10 kJ mol to 100 kJ mol
• This is a significant amount of energy
• So this bond plays an important role in deciding the properties of a sample
♦ Proteins and nucleic acids are examples
• So far in this chapter, we have seen four forces:
(i) London force (ii) Dipole-dipole force (iii) Dipole-induced dipole force (iv) Hydrogen bond
■ These four forces together are called van der Waals forces
• van der Waals was a Dutch scientist
♦ He gave a satisfactory explanation for the behaviour of gases
♦ His explanation was based on the above four forces
♦ We will learn about his works later in this chapter
• van der Waals forces are attractive forces
• But the molecules experience repulsive forces also. Details can be written in 3 steps
1. Suppose that two molecules come very close to each other
• Then the following repulsive forces will come into effect:
♦ The electron cloud of one molecule will repel the electron cloud of the other molecule
♦ The nuclei in one molecule will repel the nuclei in the other molecule
2. So we can write:
When two molecules are very close to each other, repulsive forces become predominant
3. Once the repulsive forces become predominant, we can no longer bring the molecules any closer
♦ That is why we are not able to compress solids and liquids
♦ The molecules in them are already close to each other
Thermal energy
We can write the basics of thermal energy in 9 steps:
1. Consider a sample of any substance
• It can be a sample of any substance. For example: water, carbon dioxide, sugar etc.,
2. What ever be the substance, the molecules in that sample are in motion
• Even if the sample is a solid substance, the molecules are in motion
♦ In this case, the ‘magnitude of the speed of motion’ will be very small
• Even if the sample is very cold, the molecules are in motion
♦ In this case also, the ‘magnitude of the speed of motion’ will be very small
3. If a particle is in motion, we know that, it will possess kinetic energy (K)
■ So we can write:
All molecules in the sample will have it’s own kinetic energy
♦ If the sample is a solid substance, the K possessed by each molecule will be very small
♦ If the sample is very cold, then also, the K possessed by each molecule will be very small
4. Next, we heat that sample. We know that, when heat is supplied, it’s temperature will increase
• The heat energy possessed by that sample will increase
• Heat energy is same as thermal energy
♦ So, when we heat the sample, thermal energy possessed by that sample increases
5. When heating is done, the ‘speed of motion’ of the molecules also increases
• That means, when heating is done, kinetic energy of each molecule in that sample increases
• Let us see an example. it can be written in 4 steps:
(i) Heat one end of an iron rod
♦ The end which is heated becomes hot first
(ii) The kinetic energy of the molecules at that end increases
♦ Those molecules begin to vibrate faster
(iii) When this fast vibration takes place, those molecules collide with the adjacent cold molecules
♦ During the collision, some energy is transferred to those cold molecules
(iv) Then those cold molecules become hot and they also begins to vibrate
♦ This process continues and heat reaches the other end of the rod
6. So we see that, thermal energy and kinetic energy are related
• Let there be n molecules in a sample
• Let their kinetic energies be: K1, K2, K3, . . . , Kn
• Then the total kinetic energy (K1 + K2 + K3 + . . . + Kn) is equal to the thermal energy possessed by the sample
7. We know that:
♦ In solids, the molecules have very small freedom of movement
♦ In liquids, the molecules have a greater freedom of movement than in solids
♦ In gases, the molecules have total freedom of movement
8. This gives us an idea to change the states of matter between solid, liquid and gas. It can be written in 3 steps:
(i) If we increase the thermal energy of a solid sample, the molecules in that sample will begin to vibrate faster
(ii) If we further increase the thermal energy, the molecules will become free from each other
(iii) Thus the solid will become a liquid and then a gas
9. So the question arises:
■ How much thermal energy will be needed to change the state of a sample?
The answer can be written in 5 steps:
(i) We saw that, there are some forces which try to keep the molecules together
♦ They are the inter molecular attractive forces (van der Waals forces)
(ii) So we will have to overcome these forces in order to achieve a ‘change in state’
(iii) If in a given sample, the inter molecular forces are very predominant, then:
♦ That sample will be in the solid state
♦ We will need to supply a large quantity of thermal energy to change the solid state
(iv) If in a given sample, the inter molecular forces are less predominant, then:
♦ That sample will be in the liquid state
♦ We will need to supply only a lesser quantity of thermal energy to change the liquid state to gaseous state
(v) If in a given sample, the inter molecular forces are very less predominant, then:
♦ That sample will already be in the gaseous state
Copyright©2020 Higher Secondary Chemistry. blogspot.in - All Rights Reserved
No comments:
Post a Comment